Valley International Journal Digital Library

VIJ Digital library
Articles

Rank Size Rule Analysis on the Urban Population Distribution of Indonesia for the Year 2020

PDF
  • Mohammad Sadiq Mohaqiq
Mohammad Sadiq Mohaqiq
Faculty of Economics and Business Universitas Islam Internasional ,
Published in : International Journal of Scientific Research and Management (IJSRM) , Vol. 12 No. 09 (2024)

Submission to VIJ 2024-09-23

Keywords

  • Zipf`s Law, Rank-size rule, urban hierarchy, Indonesia, population distribution, economic activity, urban studies, socioeconomic dynamics, city size distribution, sustainable urban development, infrastructure investment, urban planning, policymaking.

Copyright (c) 2024 Mohammad Sadiq Mohaqiq

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

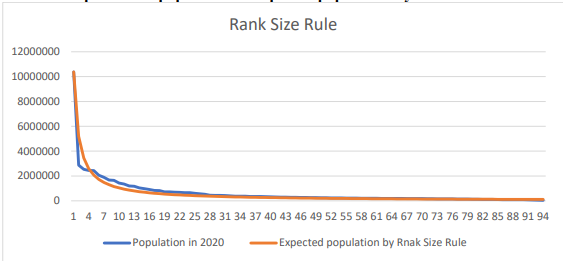
The rank-size rule which is known as Zipf``s law is an interesting research topic in the field of urban and regional studies. Conclusions are drawn based on the parameters that examine people and their economic activities in a particular city. this study aims to assess the unique urban development and population distribution of Indonesia by taking into account the complex socioeconomic system and geographically different archipelago. This study uses demographical data from various cities in Indonesia to understand the hierarchal pattern of population distribution in urban areas. Furthermore, Indonesia is an interesting case study regarding urban economics since it has a vast geographical area and contains different cultures and economic activities. The main objective of this research is to understand the population distribution and to evaluate the accuracy of the rank-size rule model in this country. This research also aimed to identify the main factors that deviate the population distribution from the rank-size rule distribution. It is particularly important to develop practical policies on the urban development issues. The minor variations of population distribution in Indonesia from the rank-size rule model are a good tool for decision-makers to allocate resources, develop infrastructure, and contribute to sustainable urban expansion. In addition, this study is significant for understanding how the urban centers developed in Indonesia and how they interact with broader socio-economic perspectives which is essential for further urban development and wise decision-making.

PDF

References

  1. Allen, G. R. (1954). The ‘Courbe des populations’, a further analysis. Bulletin of the Oxford University Institute of Statistics, 16(5/6), 179-189.
  2. Berry, B. J. (1971). City Size and Economic Development: Conceptual Syn-thesis and Policy Problems, with Special Reference to South and Southeast Asia.
  3. Berry, B. J., & Barnum, H. G. (1962). Aggregate relations and elemental components of central place systems. Journal of Regional Science, 4(1), 35-68.
  4. Berry, B. J. (1961). City size distributions and economic development. Economic development and cultural change, 9(4, Part 1), 573-588.
  5. Chen, Y., & Zhou, Y. (2003). The rank-size rule and fractal hierarchies of cities: mathematical models and empirical analyses. Environment and Planning B: Planning and Design, 30(6), 799-818.
  6. Dziewoński, K. (1972). General Theory Of Rank‐Size Distributions In Regional Settlement Systems: Reappraisal And Reformulation Of The Rank‐Size Rule. Papers in Regional Science, 29(1), 73-86.
  7. Firdaus, M., & Fitria, A. (2010). Does the rank-size rule matter in Indonesia? Determinants of the size distribution of cities. Journal of Indonesian Economy and Business (JIEB), 25(1), 114-120.
  8. Grossman, D., & Sonis, M. (1989). A reinterpretation of the Rank-Size Rule: Examples from England and the Land of Israel. In Geographical Research Forum (Vol. 9, pp. 67-108).
  9. Haran, E. G. P., & Vining Jr, D. R. (1973). A Modified Yule‐Simon Model Allowing for Intercity Migration and Accounting for the Observed Form of the Size Distribution of Cities. Journal of Regional Science, 13(3), 421-437.
  10. Hinloopen, J., & van Marrewijk, C. (2006). Comparative advantage, the rank-size rule, and Zipf's law.
  11. Ijiri, Y., & Simon, H. A. (1974). Interpretations of departures from the Pareto curve firm-size distributions. Journal of Political Economy, 82(2, Part 1), 315-331.
  12. Kosmopoulou, G., Buttry, N., Johnson, J., & Kallsnick, A. (2007). Suburbanization and the rank-size rule. Applied Economics Letters, 14(1), 1-4.
  13. Lasuen, J. R., Lorca, A., & Oria, J. (1967). City size distribution and economic growth. Ekistics, 221-226.
  14. Malecki, E. J. (1980). Growth and change in the analysis of rank—size distributions: Empirical findings. Environment and Planning A, 12(1), 41-52.
  15. Marshall, J. U. (1997). Beyond the rank-size rule: a new descriptive model of city sizes. Urban geography, 18(1), 36-55.
  16. Moore, F. T. (1959). A note on city size distributions. Economic Development and Cultural Change, 7(4), 465-466.
  17. Nota, F., & Song, S. (2012). Further analysis of the Zipf's law: Does the rank-size rule really exist?. Journal of Urban Management, 1(2), 19-31.
  18. Okabe, A. (1979). An expected rank-size rule: A theoretical relationship between the rank size rule and city size distributions. Regional Science and Urban Economics, 9(1), 21-40.
  19. Parr, J. B. (1976). A class of deviations from rank-size regularity: Three interpretations. Regional Studies, 10(3), 285-292.
  20. Rosen, K. T., & Resnick, M. (1980). The size distribution of cities: an examination of the Pareto law and primacy. Journal of urban economics, 8(2), 165-186.
  21. Rosing, K. E. (1966). A Rejection of the Zipe Model (Rank SIze Rule) in Relation to City Size. The Professional Geographer, 18(2), 75-82.
  22. Savage, S. H. (1997). Assessing departures from log-normality in the rank-size rule. Journal of archaeological Science, 24(3), 233-244.
  23. Simon, H. A. (1968). On judging the plausibility of theories. In Studies in Logic and the Foundations of Mathematics (Vol. 52, pp. 439-459). Elsevier.
  24. Singer, H. W. (1936). The “courbe des populations.” A parallel to Pareto’s Law. The Economic Journal, 46(182), 254-263.
  25. Sonis, M., & Grossman, D. (1984). Rank-size rule for rural settlements. Socio-Economic Planning Sciences, 18(6), 373-380.
  26. Steindl, J. (1978). Size distributions in economics. na.
  27. Vining Jr, D. R. (1977). The rank-size rule in the absence of growth. Journal of Urban Economics, 4(1), 15-29.